Angles of a Triangle Test-2

Angles of a Triangle Test-2 …

Angles of a Triangle Test-2 Problems

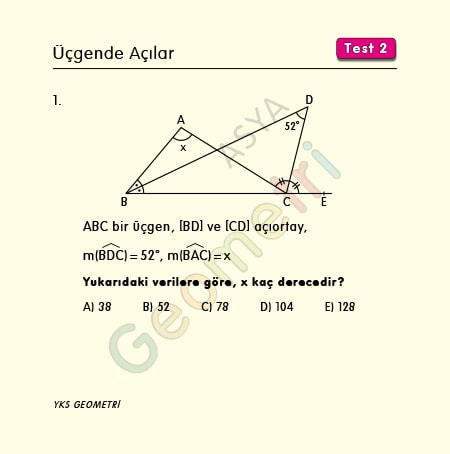
Problem 1 :

ABC is a triangle, [BD] and [CD] bisector, if m (BDC) = 52 °; How many degrees is m (BAC) = x?

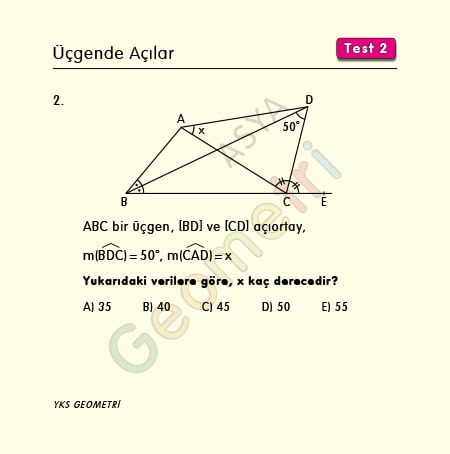
Problem 2 :

ABC is a triangle, [BD] and [CD] bisector, if m (BDC) = 50 °; How many degrees is m (CAD) = x?

Problem 3 :

In ABC triangle; D is the center of the outer tangent circle, if m (ADB) = x, m (BDC) = y, m (BAC) = 7x-5 °, m (ACB) = y-20 °; How many degrees is x?

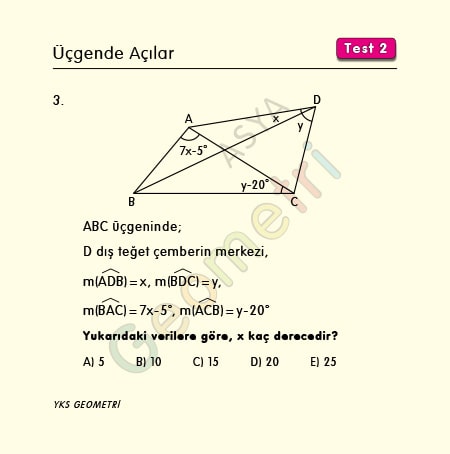
Problem 4 :

If KN parallel [CP, m (ACB) = m (BCP), | AC | = | BC |, m (CAK) = 60 °; How many degrees is m (NAB) = x?

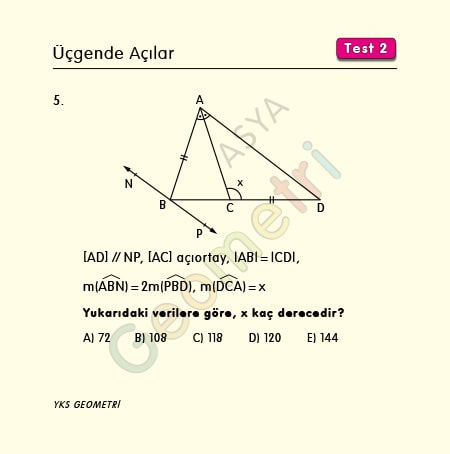
Problem 5 :

If [AD parallel NP, [AC] bisector, | AB | = | CD |, m (ABN) = 2m (PBD); How many degrees is m (DCA) = x?

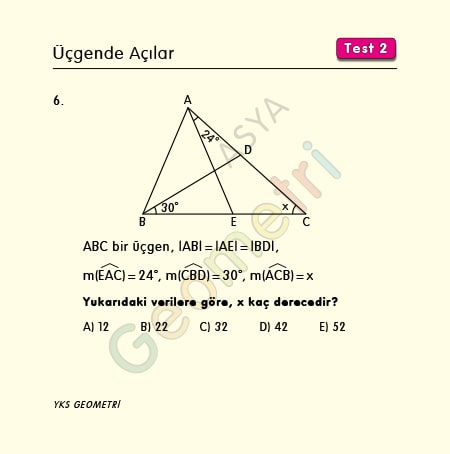
Problem 6 :

ABC is a triangle, | AB | = | AE | = | BD |, if m (EAC) = 24 °, m (CBD) = 30 °; How many degrees is m (ACB) = x?

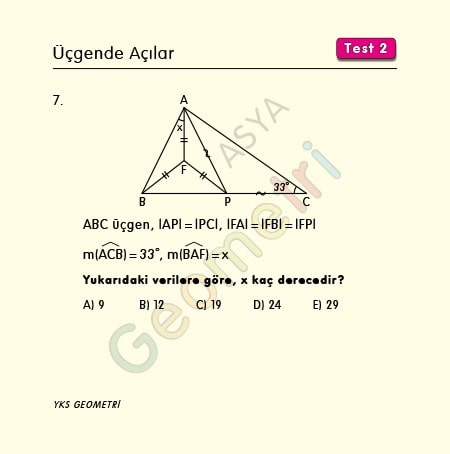
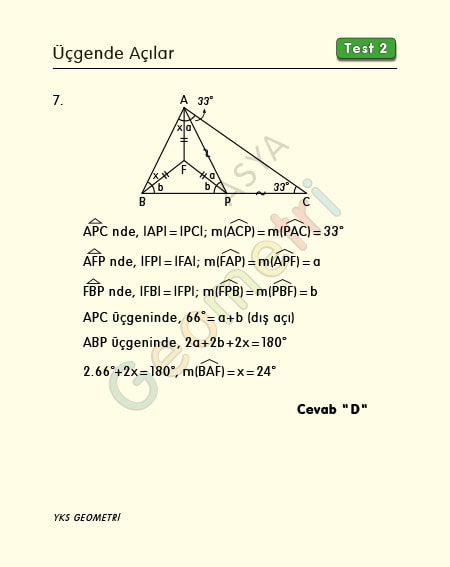
Problem 7 :

ABC triangle, if | AP | = | PC |, | FA | = | FB | = | FP |, if m (ACB) = 33 °; How many degrees is m (BAF) = x?

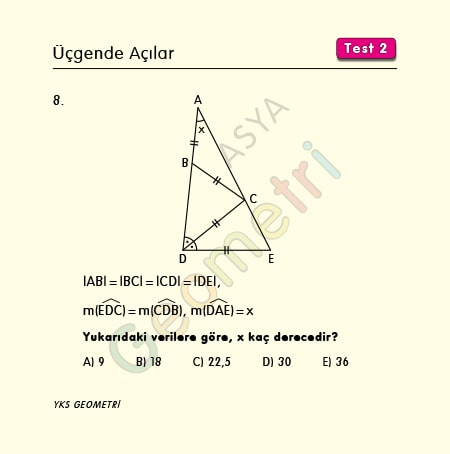
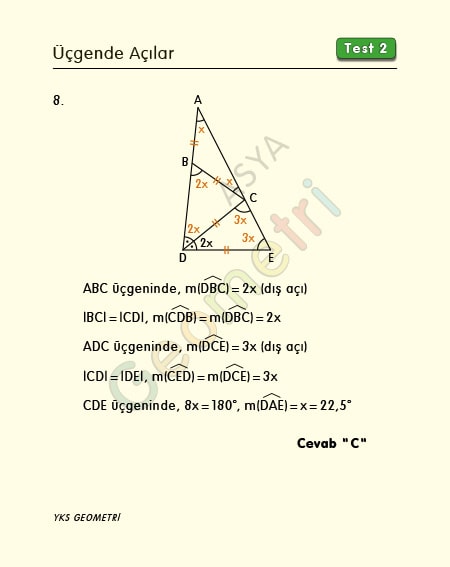
Problem 8 :

If | AB | = | BC | = | CD | = | DE |, if m (EDC) = m (CDB); How many degrees is m (DAE) = x?

Angles of a Triangle Test-2 Solutions

Solution of Problem 1 :

m (BDC) = 52 °, formed by the intersection of triangle ABC with an inner bisector and an outer bisector, will be half the angle m (BAC), so m (BAC) = 2.52 ° = 104 °.

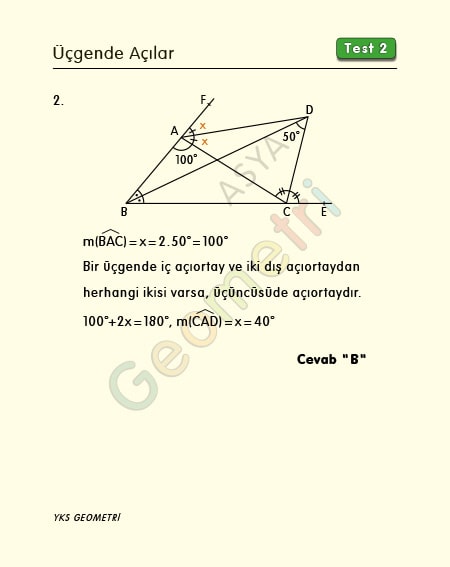
Solution of Problem 2 :

Since the triangle ABC intersects an inner bisector and an outer bisector, m (BAC) = 100 °. If there is any two of the two outer bisectors in the triangle, the third is the bisector. (Point D is the center of the outer tangent circle of triangle ABC.) In this case, m (CAD is CAD. ) = x = 40 °.

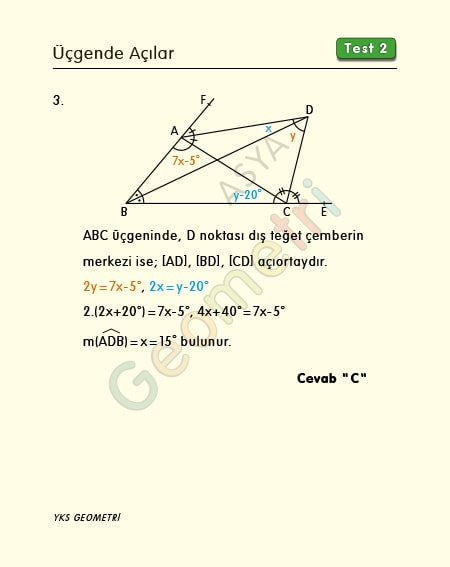
Solution of Problem 3 :

[AD], [BD], [CD] is bisector. ABC triangle; With the inner bisector of [BD], 2y = 7x-5 ° from the outer bisector of [CD], and 2x = y-20 ° from the outer bisector [AD] with the inner bisector of [BD].

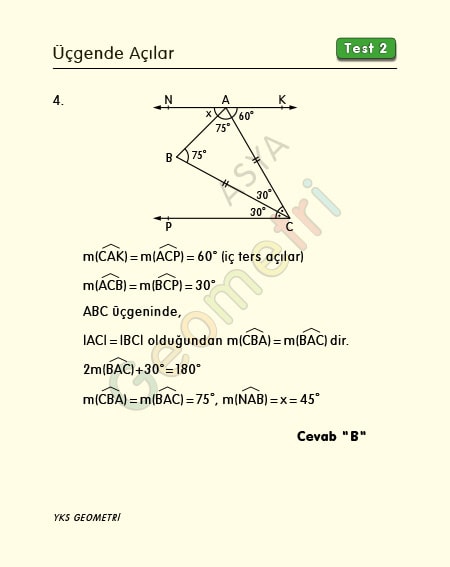
Solution of Problem 4 :

Equilateral angles from inside angles are 30 degrees. In an isosceles triangle, m (BAC) = 75 °. From the right angle x = 45 °.

Solution of Problem 5 :

If we say m (BAC) = m (CAD) = a, then m (ABN) = 2a from inside angles. If m (ABN) = 2a then m (PBD) = a. M (ADB) = a (internal inverse angle). | AC | = | CD | Since | AB | = | AC | m (ACB) = 2a (outer angle). In the triangle ABC, m (ACB) = m (CBA) = 2a. Then 5a = 180 °, a = 36 °. x = 108 °.

Solution of Problem 6 :

| AB | = | AE |; m (AEB) = m (EBA) = b + 30 °, | AB | = | BD |; m (ADB) = m (BAD) = a + 24 ° A + 2b = 120 ° in triangle ABE, 2a + b = 132 ° in triangle USA. From the solution of a system of equations with two unknowns, b = 36 °. x = 42 ° is calculated.

Solution of Problem 7 :

Since a + b = 66 ° (outer angle), 2x + 2 (a + b) = 180 ° in triangle ABP; 2x = 180 ° -132 °, x = 24 °.

Solution of Problem 8 :

From top to bottom, in the triangle, equilateral angle, outer angle, equilateral angle in triangle, equilateral angle in triangle, outer angle in triangle, equilateral angle are written. The interior angles of the triangle are added together and the angle x is calculated.

Angles of a Triangle test-2 PDF

Course Geometry offers Geometry textbook in pdf format that can be downloaded free of charge. Students can view the angles of a triangle test-2 pdf, which is important for university entrance exam, which aims to improve students’ problem solving skills.