Angles in Parallel Lines Test-2

Angles in Parallel Lines Test-2, angles in a line zigzag rule, zigzag rule, angles in a line questions and solutions, angles in a line test with answers, angles in a triangle question solution, shalwar triangle, triangles solved questions, right angles questions, triangles test …

You can review the images, from the end of the page 7.-8.-9 class in PDF format. You can download Angles on a straight line test-2 file to your computer.

Angles in Parallel lines Test-2 Problems

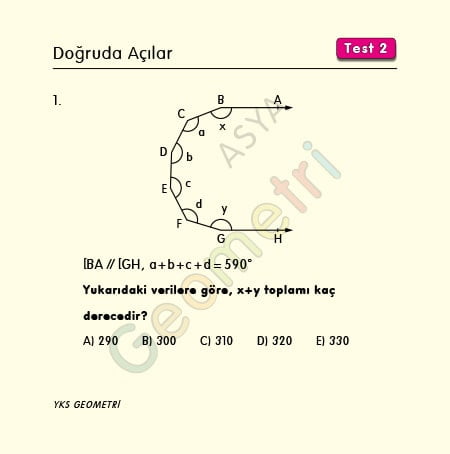
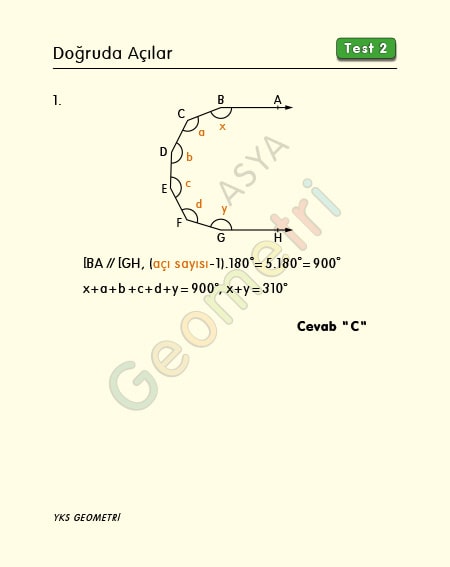
Problem 1 :

[BA parallel [GH, if a + b + c + d = 590 °; How many degrees is x + y?

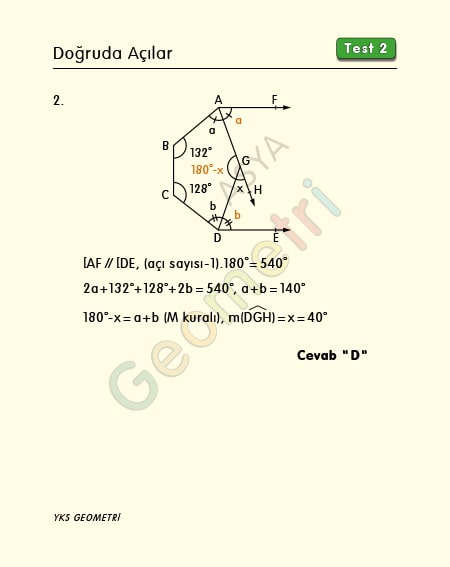
Problem 2 :

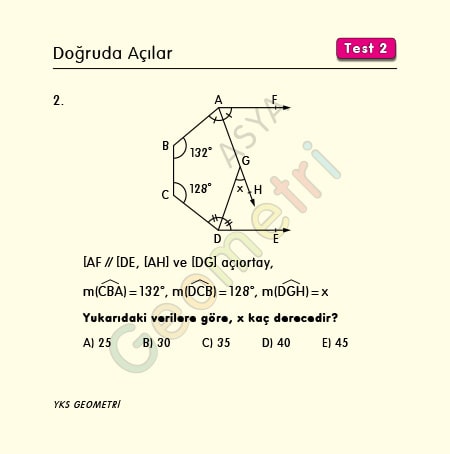
[AF parallel [DE, [AH] and [DG] bisector, if m (CBA) = 132 °, m (DCB) = 128 °; How many degrees is m (DGH) = x?

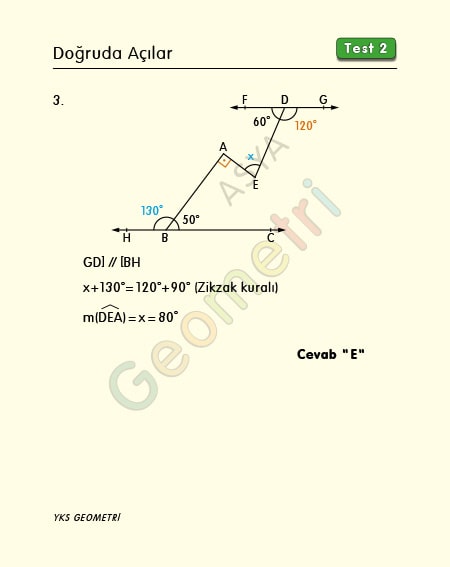
Problem 3 :

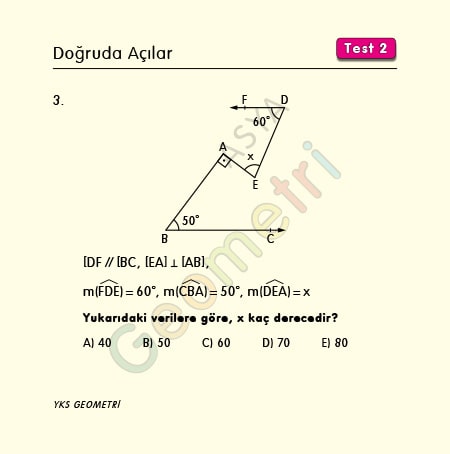
If [DF parallel [BC, [EA] perpendicular [AB], m (FDE) = 60 °, m (CBA) = 50 °; How many degrees is m (DEA) = x?

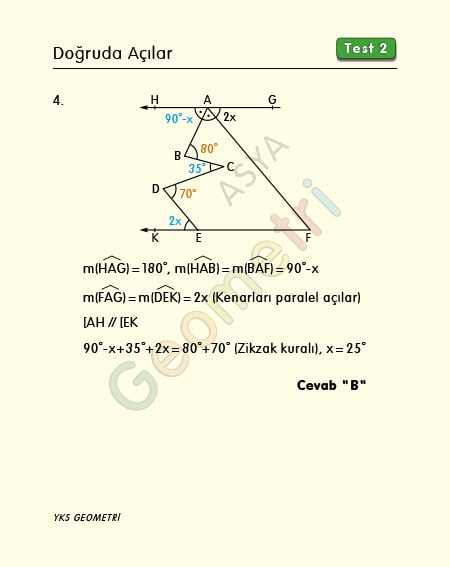
Problem 4 :

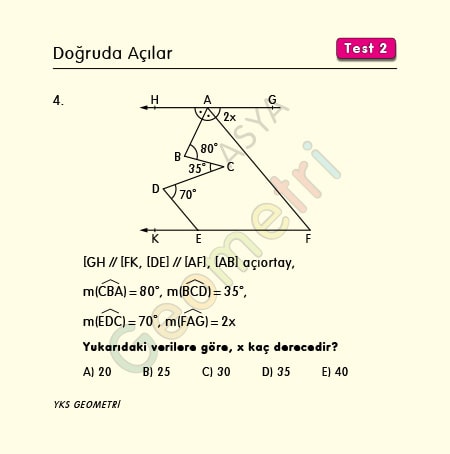
[GH parallel [FK, [DE] parallel [AF], [AB] bisector, m (CBA) = 80 °, m (BCD) = 35 °, m (EDC) = 70 °, m (FAG If) = 2x; How many degrees is x?

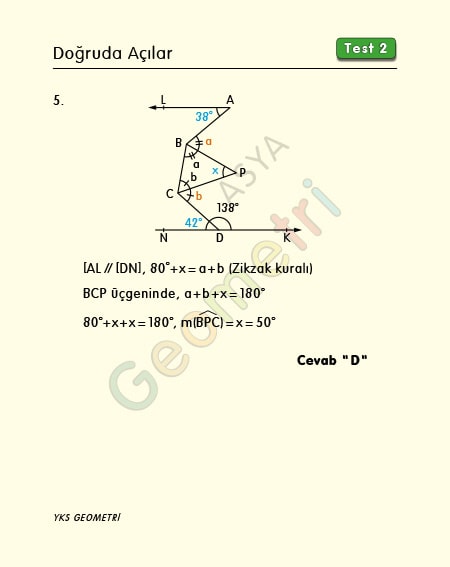
Problem 5 :

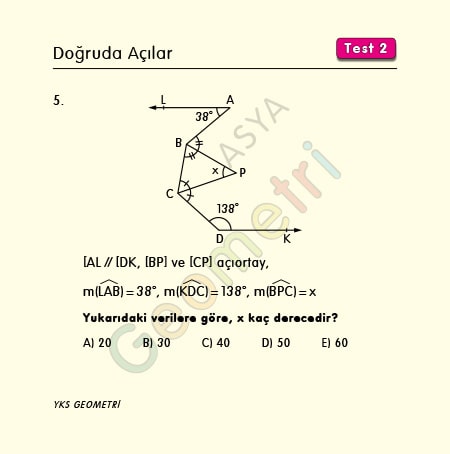
[AL parallel [DK, [BP] and [CP] bisector, if m (LAB) = 38 °, m (KDC) = 138 °; How many degrees is m (BPC) = x?

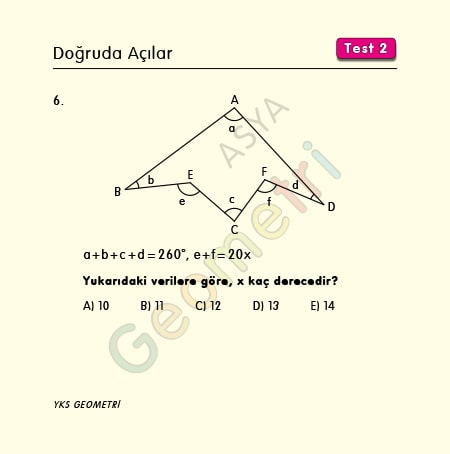
Problem 6 :

If a + b + c + d = 260 °, e + f = 20x; How many degrees is x?

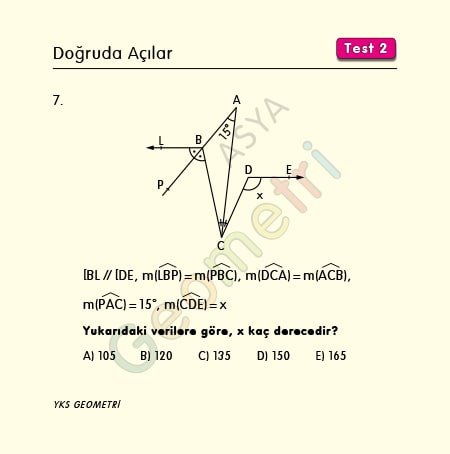
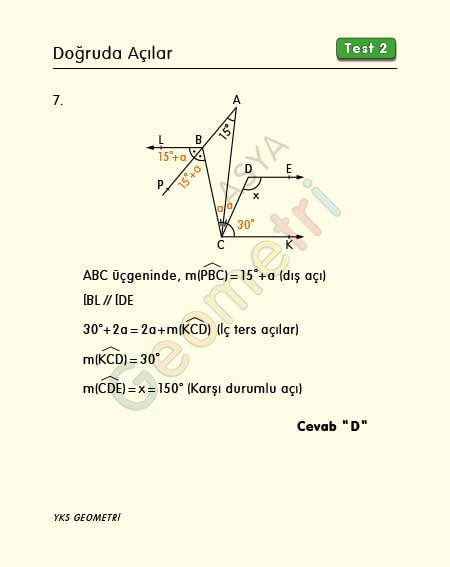
Problem 7 :

[If BL parallel [DE, m (LBP) = m (PBC), m (DCA) = m (ACB), m (PAC) = 15 °; How many degrees is m (CDE) = x?

Problem 8 :

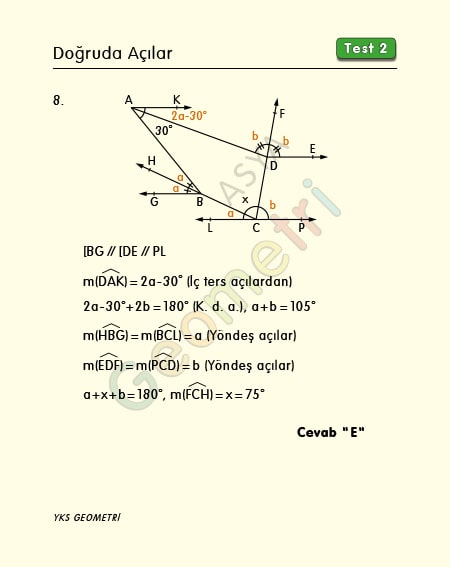
If [BG parallel [DE, m (ABH) = m (HBG), m (EDF) = m (FDA), m (BAD) = 30 °; How many degrees is m (FCH) = x?

Angles in Parallel lines Test-2 Solutions

Solution of Problem 1 :

To find the sum of the angles between two parallel lines, we add the number of vertices between the parallel lines and multiply 1 by 180 °. (angle number-1) .180 ° From the sum of angles we have obtained, we find the desired value by subtracting the value given in the question.

Solution of Problem 2 :

(angle number-1). From the formula 180 ° (see the proofs section), a + b = 140 ° is found by the rule of M, 180 ° -x = a + b, and if a + b is substituted, x = 40 ° .

Solution of Problem 3 :

Since the sum of the angles between two parallel lines on the same side is equal to the sum of the angles on the other side (zigzag rule), x + 130 ° = 120 ° + 90 °, x = 80 °.

Solution of Problem 4 :

Solution: With the sides (opposite) parallel angles, m (DEK) = 2x. The sum of the angles on the same side equals x = 25 °.

Solution of Problem 5 :

With the zigzag rule, it is 80 ° + x = a + b. If 80 ° + x is written in the triangle instead of a + b, 80 ° + 2x = 180 °, x = 50 °.

Solution of Problem 6 :

From inside angles, m (BAD) = m (ABK) = a. From Zigzag rule a + b + c + d = e + f. 260 ° = 20x, x = 13 °.

Solution of Problem 7 :

In triangle ABC, m (BPC) = 15 ° + a (outer angle). [BL // [DE with inside angles 30 ° + 2a = 2a + m (KCD), m (KCD) = 30 °. From opposite angles m (CDE) = x = 150 ° is found.

Solution of Problem 8 :

[AK // [BG; m (DAK) = 2a-30 ° (inside angles). [AK // [DE; 2a-30 ° + 2b = 180 ° (opposite-state angles) .a + b = 105 °. [BG // [CL and [DE // [CP; m (BCL) = a, m (PCD) = b (corresponding angles) .a + x + b = 180 °, 105 ° + x = 180 °, m (FCH) = x = 75 °.

Angles in Parallel lines test-2 PDF

Course Geometry offers Geometry textbook in pdf format that can be downloaded free of charge. Students can view the angles in parallel lines test-2 pdf, which is important for university entrance exam, which aims to improve students’ problem solving skills.