Special Right Triangles Test-3

Special right triangles test-3 …

Special Right Triangles Test-3 Problems

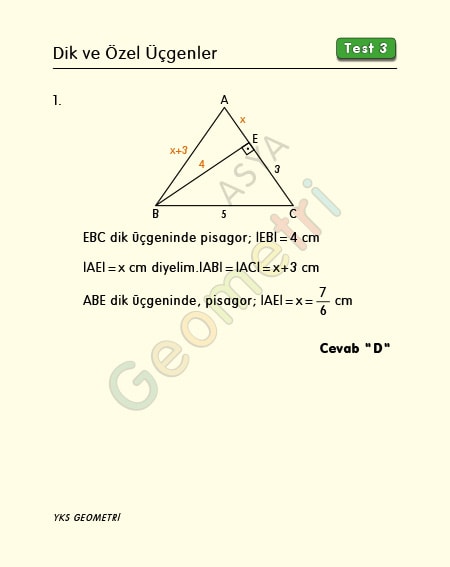
Problem 1 :

If ABC is an isosceles triangle, [BE] perpendicular [AC], | EC | = 3 cm, | BC | = 5 cm; | AC | = how many cm is x?

Problem 2 :

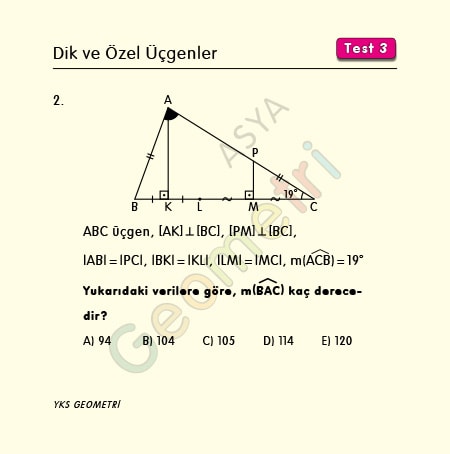
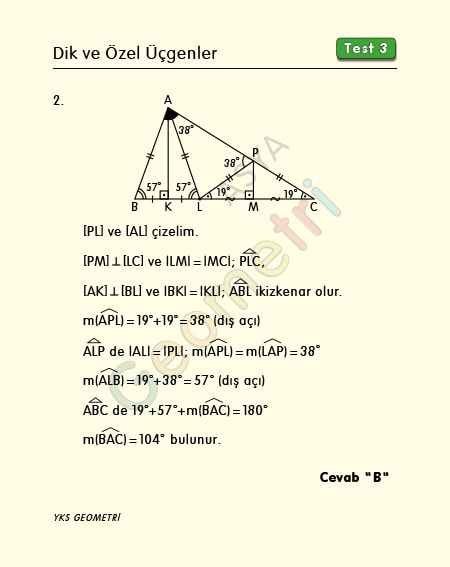
ABC is a triangle, [AK] perpendicular [BC], [PM] perpendicular [BC], | AB | = | PC |, | BK | = | KL |, | LM | = | MC |, m ( ACB) = 19 °; How many degrees is m (BAC)?

Problem 3 :

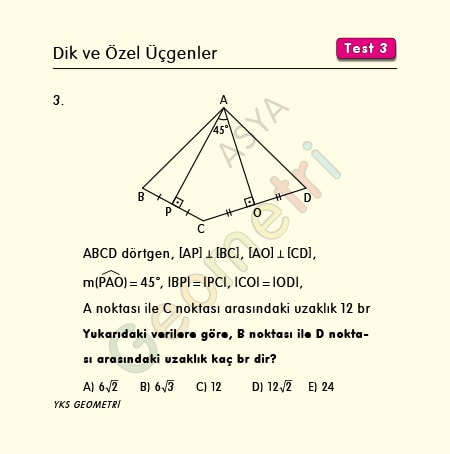
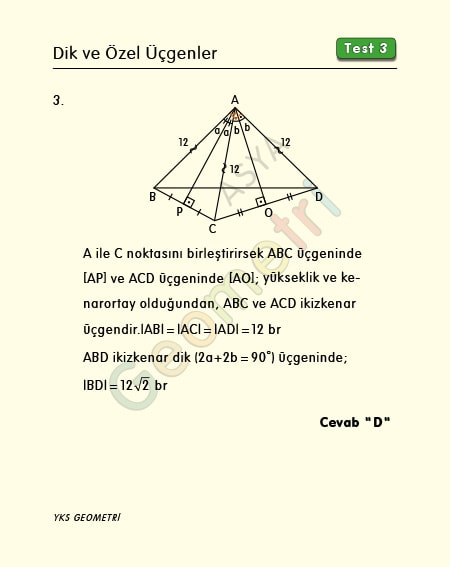
ABCD quadrilateral, [AP] perpendicular [BC], [AO] perpendicular [CD], m (PAO) = 45 ° | BP | = | PC |, | CO | = | OD |, C with point A If the distance between the point is 12 br; What is the distance between point B and point D?

Problem 4 :

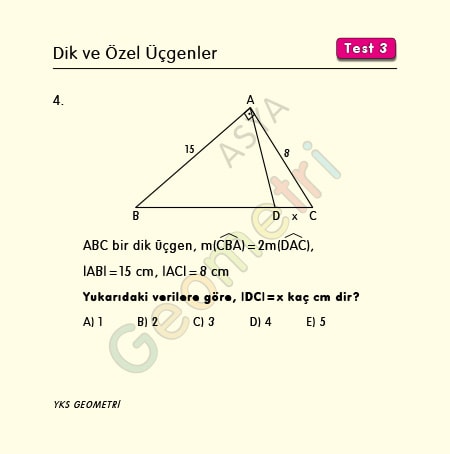
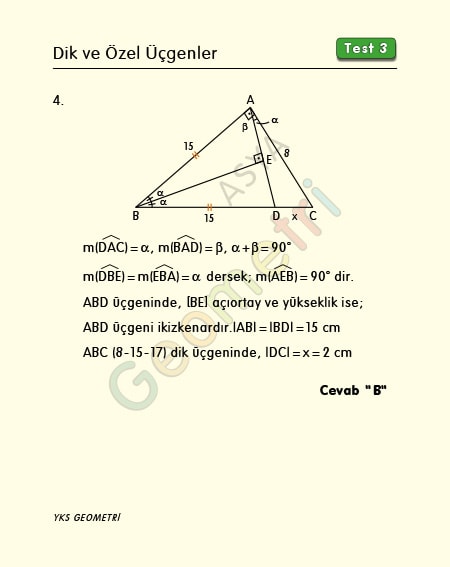
If ABC is a right triangle, m (CBA) = 2m (DAC), | AB | = 15 cm, | AC | = 8 cm; | DC | = how many cm is x?

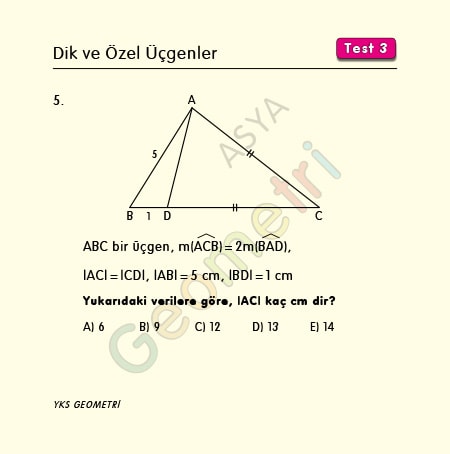
Problem 5 :

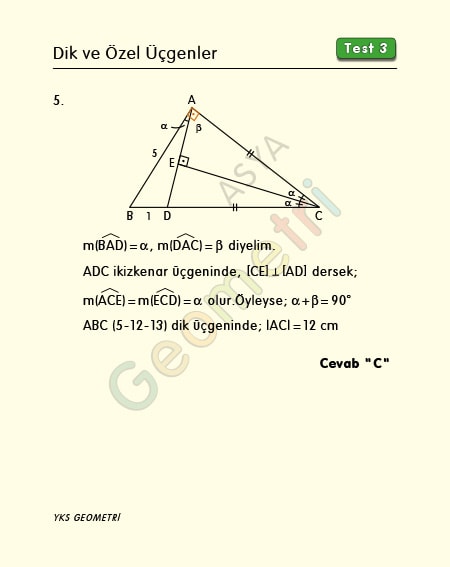
If ABC is a triangle, m (ACB) = 2m (BAD), | AC | = | CD |, | AB | = 5 cm, | BD | = 1 cm; | AC | = how many cm is x?

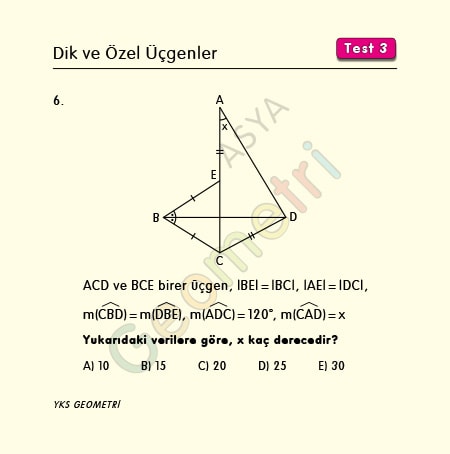
Problem 6 :

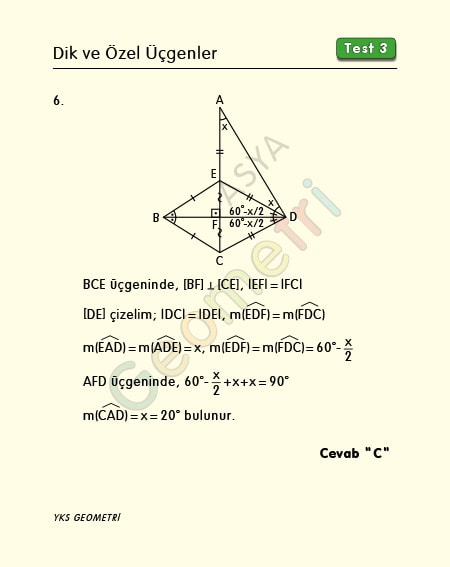
ACD and BCE are each triangle, | BE | = | BC |, | AE | = | DC |, m (CBD) = m (DBE), m (ADC) = 120 °, m (CAD) = x if; How many degrees is x?

Problem 7 :

In triangle ABC [DE] perpendicular [AC], [DF] perpendicular [AB], if m (ACB) = m (CAB) = 15 °, | DE | + | DF | = 5 cm; | AB | how many cm?

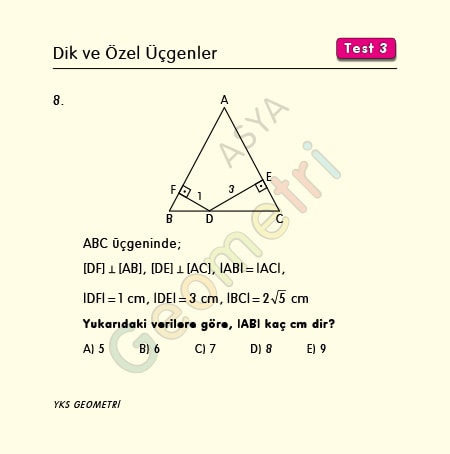
Problem 8 :

In triangle ABC [DF] perpendicular [AB], [DE] perpendicular [AC], | DF | = 1 cm, | DE | = 3 cm, | BC | = 2 root 5 cm; | AB | how many cm?

Special Right Triangle Test-3 Solutions

Solution of Problem 1 :

EBC is the right triangle (3-4-5). If we say | AE | = x in ABC triangle, it is | AB | = x + 3. In right triangle ABE, x = 7/6 cm from Pythagoras relation.

Solution of Problem 2 :

If we connect the point L with the point P in ABC triangle, isosceles triangles are formed. The angle A is found by writing the angles of the isosceles triangles.

Solution of Problem 3 :

In two isosceles triangles that are formed, if we combine points A and C, the sum of the vertex angles of isosceles triangles is 2a + 2b, which is 90 degrees, since the heights are at the same time isosceles.

Solution of Problem 4 :

In the US triangle, the perpendicular descending from corner B becomes an isosceles triangle since the angles will be bisector when the angles are lettered. Since ABC is a right triangle, x = 2 cm.

Solution of Problem 5 :

In an isosceles triangle, the height of the base is the bisector. If the angles are written, ABC is a right triangle and from the Pythagorean equation | AC | = 12 cm.

Solution of Problem 6 :

In an isosceles triangle BCE, the bisector becomes both the height and the median at the same time. If we connect E with point D, in DCE triangle the triangle becomes isosceles since the median and the height are the same line. (There are two isosceles adjoined to the base, which is called the deltoid) In the AFD triangle, the angle x is 20 ° from the sum of internal angles.

Solution of Problem 7 :

In an isosceles triangle, the sum of the perpendiculars drawn from a point taken on the base to the equilateral sides is equal to the height of the identical sides. If the side opposite 30 ° in a right triangle is 5 cm, then the hypotenuse will be 2 times the side opposite to 30 °, so the answer is 10 cm

Solution of Problem 8 :

In an isosceles triangle, the sum of the posts lowered to equal sides from a forehead point on the base is equal to a height of equal sides. | BH | = 3 + 1 = 4 cm. If we say | AH | = x, then | AB | = x + 2. In the triangle ABH, from the Pythagorean equation, | AB | = | AC | = 5 cm.

Special Right Triangles Test-3 PDF

Course Geometry offers Geometry textbook in pdf format that can be downloaded free of charge. Students can view the special right triangles test-3 pdf, which is important for university entrance exam, which aims to improve students’ problem solving skills.