Special Right Triangles Test-5

Special Right Triangles Test-5 Problems
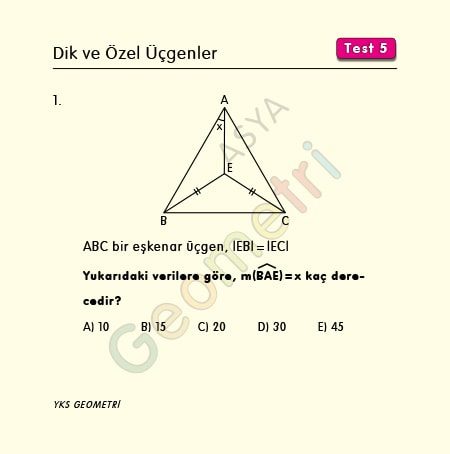
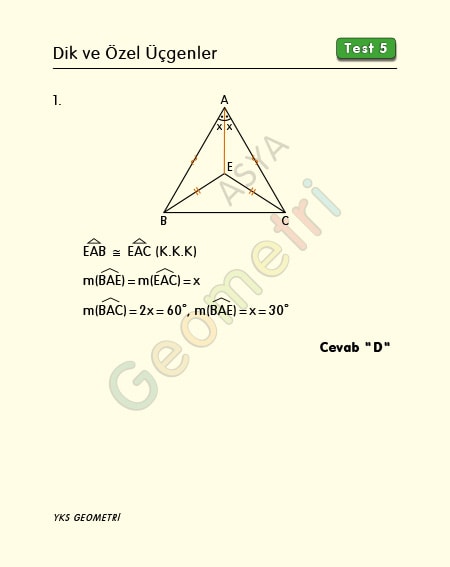
Problem 1 :
ABC is an equilateral triangle, | EB | = | EC | if; m (BAE) = How many degrees is x?
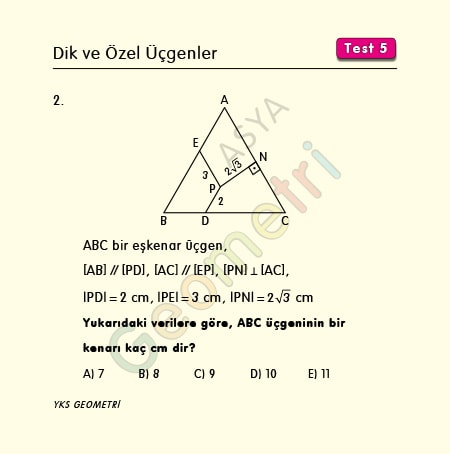
Problem 2 :
ABC is an equilateral triangle, [AB] parallel [PD], [AC] parallel [EP], [PN] perpendicular [AC], | PD | = 2 cm, | PE | = 3 cm, | PN | = 2 root if 3 cm; How many cm is a side of triangle ABC?
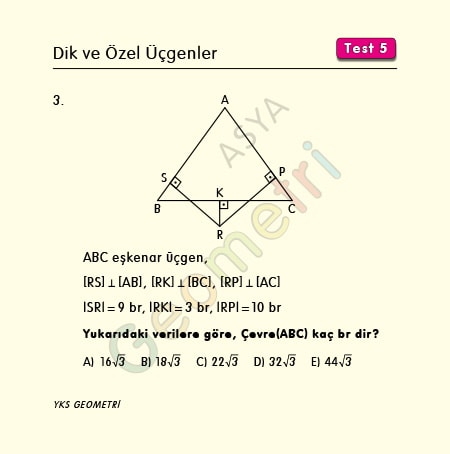
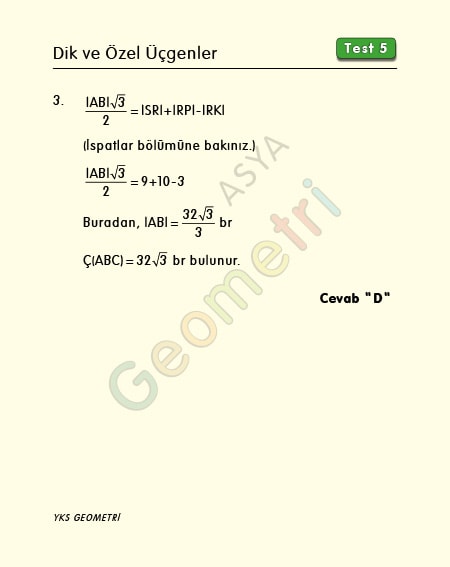
Problem 3 :
ABC equilateral triangle, [RS] perpendicular [AB], [RK] perpendicular [BC], [RP] perpendicular [AC], | SR | = 9 br, | RK | = 3 br, | RP | = If 10 br; What is the perimeter of triangle ABC?
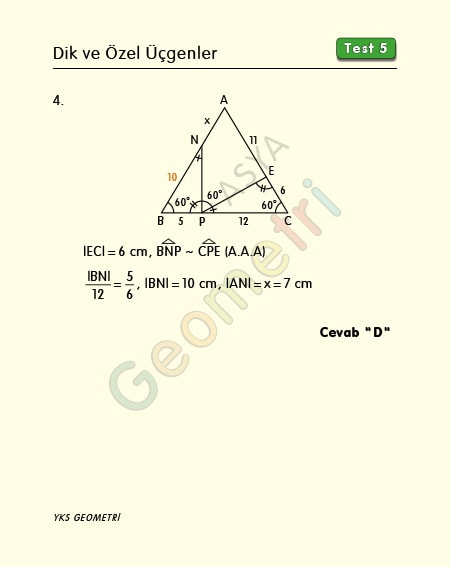
Problem 4 :
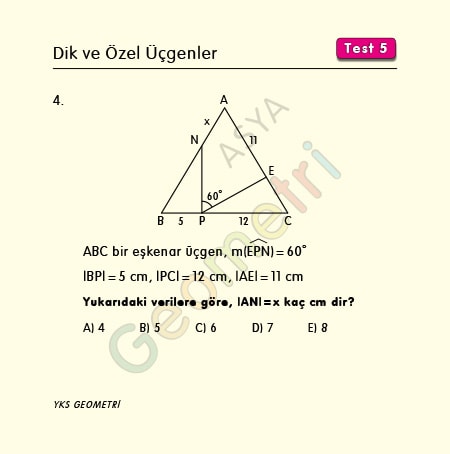
f ABC is an equilateral triangle, m (EPN) = 60 °, | BP | = 5 cm, | PC | = 12 cm, | AE | = 11 cm; | AN | = how many cm is x?
Problem 5 :
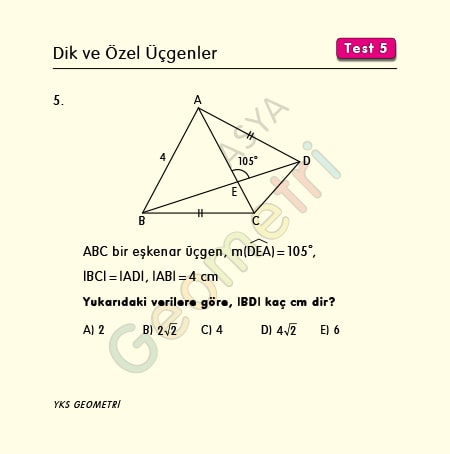
ABC is an equilateral triangle, if m (DEA) = 105 °, | BC | = | AD |, | AB | = 4 cm; | BD | how many cm?
Problem 6 :
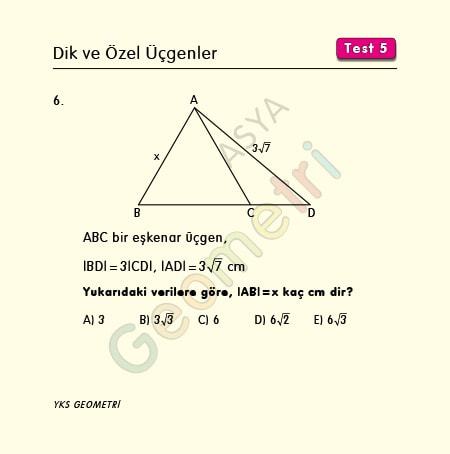
If ABC is an equilateral triangle, | BD | = 3 | CD |, | AD | = 3 root7 cm; | AB | = how many cm is x?
Problem 7 :
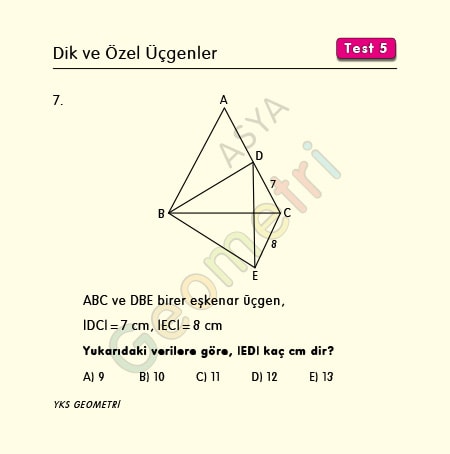
If ABC and DBE are equilateral triangles, | DC | = 7 cm, | EC | = 8 cm; | ED | how many cm?
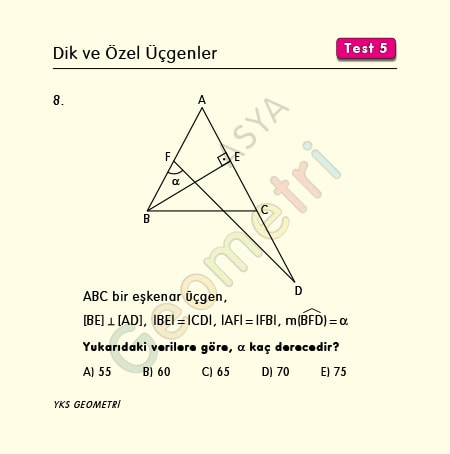
Problem 8 :
If ABC is an equilateral triangle, [BE] perpendicular [AD], | BE | = | CD |, | AF | = | FB |, m (BFD) = α; How many degrees is α?
Special Right Triangle Test-4 Solutions
Solution of Problem 1 :
Since the three sides of the triangles in an equilateral triangle are equal ( sss ), the triangles are equal. Accordingly, it is 2x = 60 °, x = 30 °.
Solution of Problem 2 :
The sum of the parallels drawn to the sides from any point taken inside the equilateral triangle is equal to one side of the equilateral triangle. For proof, see the geometry question bank pdf page.

Solution of Problem 3 :
We proved the formula for the ABC equilateral triangle in the figure in our book .
Solution of Problem 4 :
| EC | = 17-6 = 11 cm, if the angles are written instead, the length x length is 7 cm from the analogy of the two triangles aaa .
Solution of Problem 5 :
m (EBA) = 45 °, | BC | = | AD | and | BC | = | AB | is | AD | = | AB | Since m (ADB) = 45 °. Since the right sides of the US right triangle are 4 cm, the hypotenuse (| BD |) is 4 root2 cm.
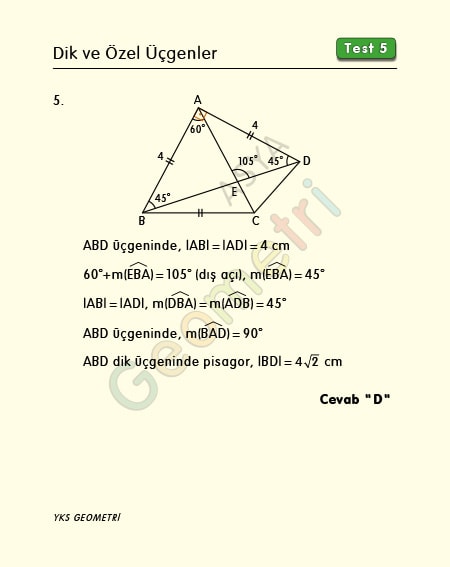
Solution of Problem 6 :
In equilateral triangle ABC, the height is median. So | BN | = | NC | is. | BD | = 3 | CD | is | BN | = | NC | = | CD | happens. In the right triangle, x = 6 cm from the Pythagorean equation.
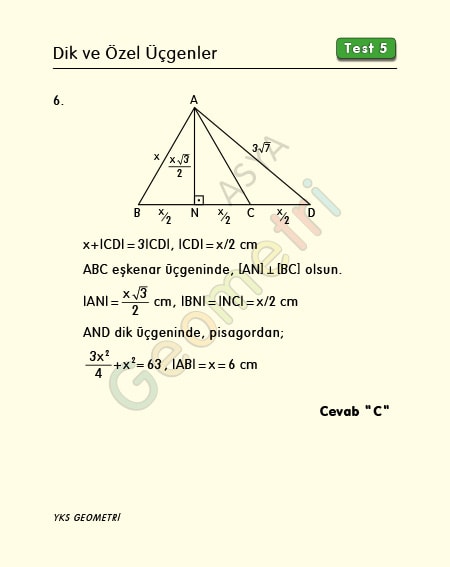
Solution of Problem 7 :
Since the two triangles are equal ( sas ), m (BCE) = 60 °. Let’s write the cosine theorem, since two sides and one angle of the triangle are known. x² = 7² + 8²-2.7.8.cos120 hence the value of x is 13 cm.

Solution of Problem 8 :
In triangle ABC | AF | = | FB | Since the height we will draw from point C to the edge of AB is perpendicular at point F. In an equilateral triangle, the heights are equal. Therefore | BE | = | CF | Then | CF | = | CD | happens. Since the base angles in an isosceles triangle with a peak angle of 150 degrees will be 15 °, the measure of the alpha angle is 90 ° -15 ° = 75 °.
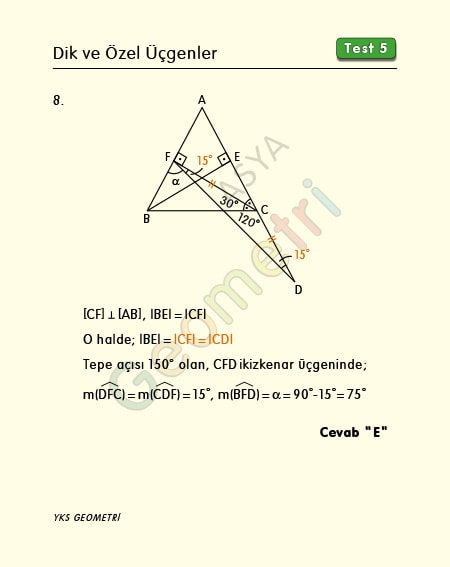

Special Right Triangles Test-5 PDF
Course Geometry offers Geometry textbook in pdf format that can be downloaded free of charge. Students can view the special right triangles test-5 pdf, which is important for university entrance exam, which aims to improve students’ problem solving skills.